Field at Work
- Potential and potential energy
- Potential gradient
- Potential difference
- Escape speed
- Orbital motion, orbital speed and orbital energy
- Forces and inverse-square law behavior

Orbital Motion
Orbital Speed
- Orbital velocity is the minimum velocity at which a body must move to maintain a given orbit, represented by the equation below.
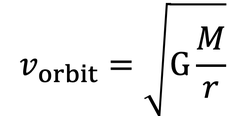
Equation 1: Orbital Velocity
This is because for a planet orbiting around another object, its centripetal force is equal to its net force of gravitational attraction force, so you can rearrange the equation and calculate its orbital speed.

By calculating its orbital speed, we can also express its kinetic energy, shown by the equation below.

- If the total energy is positive, the object will follow a hyperbolic path and never return.
-
If the total energy is zero, the object will follow parabolic path to infinity, where it will just about stop. It will never return.
-
If the total energy is negative, the object will go into a circular or elliptical orbit (or crash into the planet if the launching speed is too low).
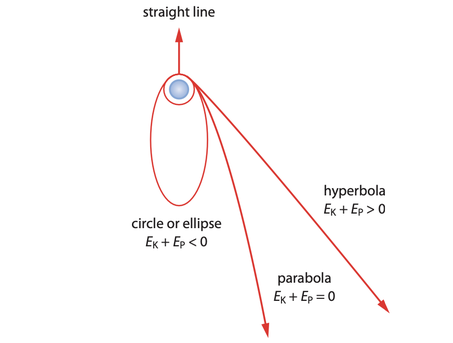
Orbital Energy

When an object is moved from a lower to a higher orbital, its total energy increases, gravitational energy increases by becoming more positive, and kinetic energy decreases, meaning that the object's speed also decreases. (This is frequently tested in paper 1) (Take a quiz)
Escape Velocity
- Escape velocity the minimum velocity that a moving body(such as a rocket) must have to escape from the gravitational field of a celestial body (such as the earth) and move outward into space.
- When an object's escapes from a celestial object, we assume the object has a velocity of zero since it is very far away from the body, so its initial kinetic energy equals to its final gravitational energy (conservation of energy).
Equating the expressions for the energy:
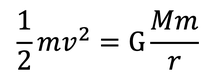
Rearranging and simplifying, we obtain:
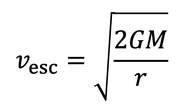
Equation 2: Escape Velocity
Note: It's important to understand the link between different quantities and how they can be expressed in terms of another. For example, escape velocity is equal to the square root of two multiply by the square root of gravitational potential.
Weightlessness
Weightlessness is the complete or near-complete absence of the sensation of weight. For an astronaut in space, it receives a gravitational attraction force by earth and normal force from space floor, assuming that he or she is in a space shift.
By using Newton's second law, we get:
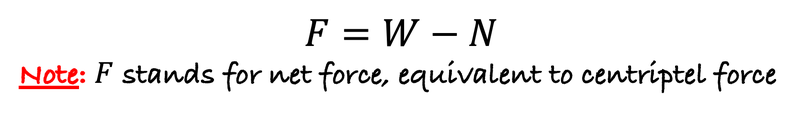
Solving for normal force, we obtained the equation below
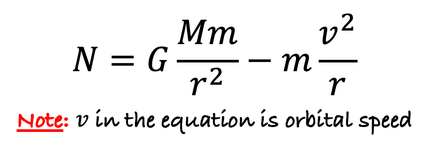
Plug in the equation of orbital speed, we normal force is equal to zero
