Sequence and Series
Learning Objectives
- Arithmetic Sequence
-
- Finding terms in an arithmetic sequence
-
- Arithmetic Series
- Sum of arithmetic series
- Geometric Sequence
- Finding terms in a geometric sequence
- Geometric Series
- Sum of finite geometric series
- Sum of infinite geometric series
- Financial Application
- Compound interest
- Annual/Quarter/Monthly interest


Important Concepts
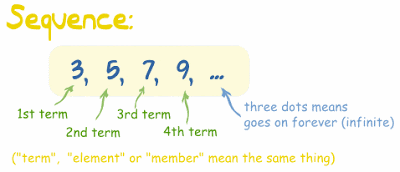
- Sequence: A sequence is a list of numbers that written in a defined order, ascending order, following a specific rule.
- Term: Each of the number making up a sequence is known as a term. Sometime a sequence is also know as a progression.
- Finite Sequence: A sequence is finite if it has a limited number of terms and infinite if it does not
- Infinite Sequence: An infinite sequence is an endless progression of discrete objects, especially numbers. A sequence has a clear starting point and is written in a definite order.
- Series: Series refers to the sum of the terms in a sequence
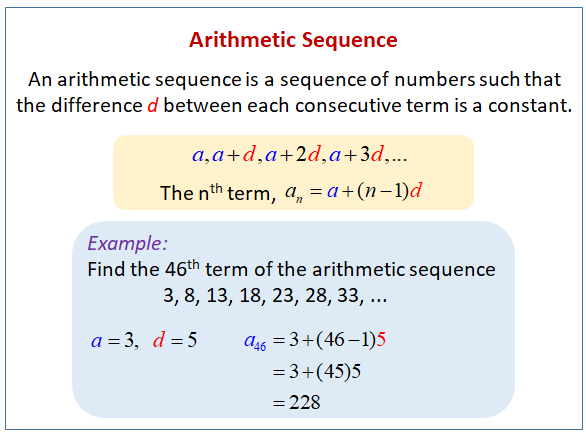
- Arithmetic Series: The sum of terms in a sequence that is added by a common difference
- Geometric Series: The sum of terms in a sequence that is multiple by a common a ration
- Harmonic Series: The infinite sum of of the reciprocals of positive integers.
- Finite Series: A finite series is given by all the terms of a finite sequence, added together. An infinite series is given by all the terms of an infinite sequence, added together.
- Infinite Series: A finite series is given by all the terms of a finite sequence, added together. An infinite series is given by all the terms of an infinite sequence, added together.

Arithmetic Sequence

By <a href="//commons.wikimedia.org/wiki/User:V.pantaloni" title="User:V.pantaloni">Vincent Pantaloni</a> - <span class="int-own-work" lang="en">Own work</span>, CC BY-SA 4.0, Link
Geometric Sequence
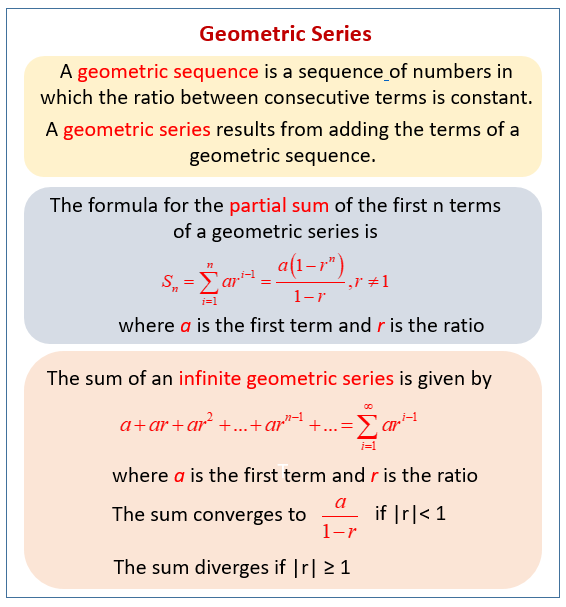
The image demonstrates geometric series from a geometric view instead of an algebraic perspective.

- By en:User:Jim.belk (original); Pbroks13 (talk) (redraw) - en:Image:GeometricSquares.png, Public Domain, https://commons.wikimedia.org/w/index.php?curid=4304109
- https://www.onlinemathlearning.com/geometric-series.html
How to Derive the Formula?


Financial Application of Geometric Series
POP QUIZ!!!
